Does quantum mechanics need imaginary numbers?

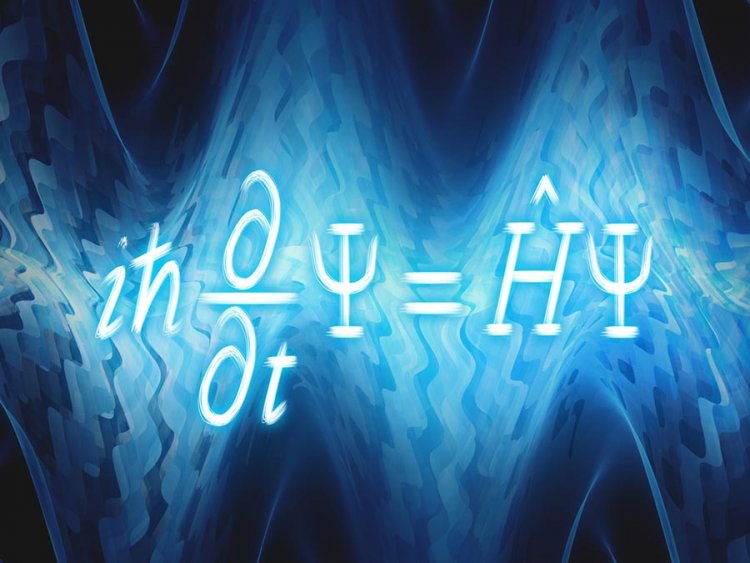
The quantum world is inherently uncertain. A quantum state doesn’t contain enough information to prescribe the outcome of every possible measurement on the state; rather, for most measurements, it offers only a probability distribution among the possible outcomes.

It’s natural to wonder whether the uncertainty means that quantum mechanics is an incomplete theory. Could it be supplemented by a system of local hidden variables that do, in fact, preordain every measurement outcome? Thanks to the work of John Bell and others, that idea has been laid to rest. Experiments can be designed that distinguish quantum predictions from those of any theory of local hidden variables. When the experiments are performed, a quantum theory emerges triumphant every time.

Another way that quantum theory challenges classical intuition is in its inclusion of imaginary numbers. Its observable quantities and probability distributions are all, by necessity, real numbers—but the underlying wavefunctions and quantum states usually involve the unphysical number i, the square root of −1. But are imaginary numbers a necessary feature of the theory, or are they just an artifact of its formulation, so that an all-real formulation could be found that works just as well?

That’s a subtle question. One can always represent the space of complex numbers as a two-dimensional space of real numbers, with one dimension representing a complex number’s real part and the other the imaginary part. But quantum states themselves are represented mathematically as multidimensional spaces—and as it turns out, the combination of dimensions upon dimensions significantly complicates the mathematics of how quantum systems interact.

In their new work, Miguel Navascués of the Austrian Academy of Sciences in Vienna and colleagues refine the question: Is it possible to formulate a version of quantum theory that retains some of the key mathematical properties of standard quantum theory—in particular, that the composition of two systems is represented by their tensor product—but that uses only real numbers? They found that the answer is no.

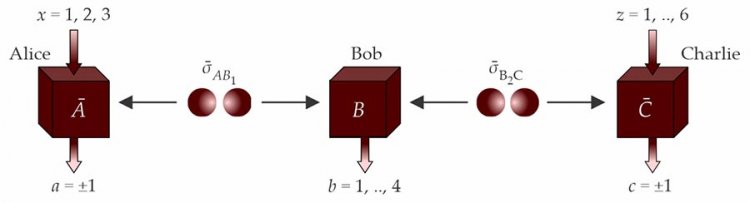
Credit: Adapted from M.-O. Renou et al., Nature 600, 625 (2021)

Navascués and colleagues devised an experiment, sketched in the figure, for which any such real-valued theory predicts different results than standard quantum theory. The experiment is only a little more involved than the one used to test Bell’s inequality: Of two pairs of entangled particles, Bob receives one of each, and the other two go to Alice and Charlie. Bob makes a joint measurement on his particles, and Alice and Charlie each choose from a few different measurements to make on theirs. As with Bell’s inequality, theories can be distinguished by the predictions they make about the correlations among the measurement outcomes.

Unlike Bell’s inequality, however, calculating the expected correlations is extremely difficult. To find an upper bound for real-valued theories, Navascués and colleagues embarked on a computation so involved that they ran out of computer memory. They had to make do with a looser bond than they were hoping for.

Still, in the time since the proposal was made public last January, two groups carried out the experiment—and both found results in favor of the standard complex-valued quantum theory. Future students of quantum mechanics, it seems, will have no choice but to grapple with the mathematics of imaginary numbers. (M.-O. Renou et al.,